 Approaches to Pilot Modeling
Approaches to Pilot Modeling Approaches to Pilot Modeling
Approaches to Pilot Modeling
(in progress)
Pilot models have many forms depending upon the flight task, vehicle type, and application. The following is a continually evolving list of topics and methods that are useful in constructing pilot models.
______________________________________________________________
Pilot model functional elements
The two primary elements of a pilot model are the decision-making and controller functions. The role of the latter is to set the piloting technique and control strategy appropriate to the task segment. The role of the former is to decide when to switch from one controller form to the next depending upon flight task demands.
An example of how the functional elements are arranged is shown below:
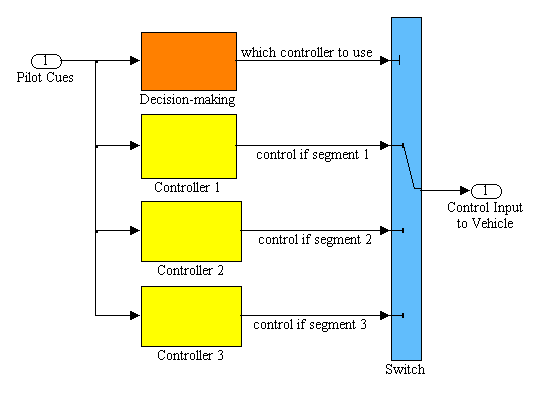
Depending upon the pilot's interpretation of cues (time, position, speed, etc.) there will be a decision to shift from one control technique or strategy to the next, depending upon the segment. Decision making is largely a logic function.
Each controller produces a control input command that is appropriate to each task segment.
______________________________________________________________
In performing any given flight task, the human pilot has a choice of control strategies and techniques--only a limited range of these will succeed in achieving a given level of performance.
How do we discover that range?
Role of the crossover model of manual control
There are several ways to construct a viable controller for each axis and level of control. This is one such way that is simple, direct, has a demonstrated application to manual control, and has been used often.
The crossover model means simply that the combined open-loop pilot-vehicle resembles a "K/s" transfer function with the numerical value of K equal to the closed-loop bandwidth, wc.
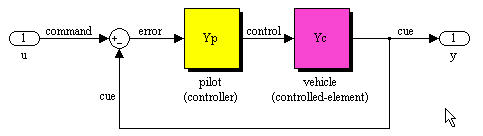
This means that the pilot must adapt a control strategy that complements the vehicle response by producing the K/s ideal. In terms of the above block diagram, Yp·Yc = wc/s.
For example, if the vehicle exhibits a "rate" response, the pilot can simply supply a pure gain between the response error and vehicle flight control. This is generally the easiest piloting situation.
If the vehicle response is a pure gain then the pilot must adopt a strategy akin to an integrator. While not ideal this is a fairly easy process.
Going in the other direction from the ideal K/s, if the vehicle response is an "acceleration" response then the pilot must control with sharp impulse inputs. This is substantially more difficult than the pure-gain ideal strategy.
The net result of the crossover model is a closed-loop system response that resembles a lag with a time constant equal to the inverse of wc. For a 2 rad/sec wc a step command by the pilot produces a rise time to 63% in 1/2 sec.
--------------------------------------------------------------------------